Keywords
Abstract
Purpose: This study presents a novel application of the semianalytical residual power series method to investigate a one-dimensional fractional anisotropic curvature equation describing the human cornea, the outermost layer of the eye. The fractional boundary value problem, involving the fractional derivative of curvature, poses challenges that conventional methods struggle to address.
Methods: The analytical results are obtained by utilizing the simple and efficient residual power series method. The proposed method is accessible to researchers in all medical fields and is extendable to various models in disease spread and control.
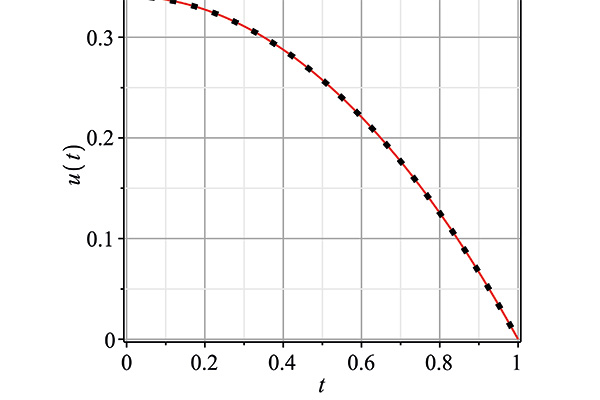
Results: The derived solution is a crucial outcome of this study. Through the application of the proposed method to the corneal shape model, an explicit formula for the curvature profile is obtained. To validate the solution, direct comparisons are made with numerical solutions for the integer case and other analytical solutions available in the literature for the fractional case.
Conclusion: Our findings highlight the potential of the proposed method to significantly contribute to the diagnosis and treatment of various ophthalmological conditions.
References
Amiri I, Yupapin P, Rashed A. Mathematical model analysis of dispersion and loss in photonic crystal fibers. Journal of Optical Communications, 2023;44, 139–144. doi: 10.1515/joc-2019-0052.
Ghasemi P, Goodarzian F, Gunasekaran A, AbrahamA. A bi-levelmathematical model for logistic management considering the evolutionary game with environmental feedbacks. The international journal of logistics management, 2023;34, 1077–1100. doi: 10.1108/ijlm-04-2021-0199.
Shanthi R, Devi M, Abukhaled M, Lyons M, Rajendran L. Mathematical modeling of pH-based potentiometric biosensor using Akbari-Ganji method. International Journal of Electrochemical Science. 2022;17, 220349. doi: 10.20964/2022.03.48.
Abukhaled M, Guessoum N, Alsaeed N. Mathematical modeling of light curves of RHESSI and AGILE terrestrial gamma-ray flashes. Astrophysics and Space Science, 2019;364, 1–16. doi: 10.1007/s10509-019-3611-3.
Saravanakumar S, Eswari A, Rajendran L, Abukhaled M. A mathematical model of risk factors in HIV/AIDS transmission dynamics: observational study of female sexual network in India. Appl. Math. Inf. Sci. 2020;14, 967–976. doi: 10.18576/amis/140603.
Pandolfi A. Cornea modelling. Eye and Vis, 2020;7(2): doi: 10.1186/s40662-019-0166-x.
Pinsky PM, Holliday K. Finite element modeling of metabolic species transport in the cornea with a hydrogel intrastromal inlay. Invest Ophthalmol Vis Sci, 2015;56(7): 1131.
Pandolfi A, Manganiello F. A model for the human cornea: Constitutive behavior and numerical analysis. Biomech Model Mechanobiol, 2006;5(4): 237–246.
Montanino A, Gizzi A, Vasta M, Angelillo M, Pandolfi A. Modeling the biomechanics of the human cornea accounting for local variations of the collagen fibril architecture. ZAMM - Zeitschrift fur Angewandte Mathematik und Mechanik, 2018;98, 2122–2134.
Montanino A, Angelillo M, Pandolfi A. A 3D fluid-solid interaction model of the air puff test in the human cornea. J Mech Behav Biomed Mater, 2019;94, 22–31.
Pinsky PM, Datye DV. A microstructurally-based finite element model of the incised human cornea. Journal of Biomechanics, 1991;24(10): 907–922.
Pinsky PM, DatyeDV. Numerical modeling of radial, astigmatic, and hexagonal keratotomy. Refractive & Corneal Surgery, 1992;8(2): 164–172.
Pandolfi A, Holzapfel GA. Three-dimensional modeling and computational analysis of the human cornea considering distributed collagen fiber orientation. Journal of Biomechanical Engineering, 2008;130(6): 061006. doi: 10.1115/1.2982251.
Pandolfi A, Fotia G, Manganiello F. Finite element analysis of laser refractive corneal surgery. Engineering Computations, 2009;25, 15–24.
Abro K, Atangana A, Gómez-Aguilar J. A comparative analysis of plasma dilution based on fractional integro-differential equation: an application to biological science. Int J Modell Simul, 2023;43, 1–10. doi: 10.1080/02286203.2021.2015818.
Ali Z, Rabiei F, Hosseini K. A fractal–fractional-order modified Predator–Prey mathematical model with immigrations. Math Comput Simul, 2023;207, 466–481. doi: 10.1016/j.matcom.2023.01.006.
Azeem M, Farman M, Abukhaled M, Nisar K, Akgul A. Epidemiological analysis of human liver model with fractional operator. Fractals, 2023;28, 2340047. doi: 10.1142/s0218348x23400479.
Shoaib M, Abukhaled M, Kainat S, Nisar K, Raja M, Zubair G. Integrated Neuro-Evolution-Based Computing Paradigm to Study the COVID-19 Transposition and Severity in Romania and Pakistan. Int J Comput Intell Syst. 2022;15, 80. doi: 10.1007/s44196-022-00133-1.
Vieru D, Fetecau C, Shah N, Yook S. Unsteady natural convection flowdue to fractional thermal transport and symmetric heat source/sink. Alex Eng J, 2023;64, 761–770. doi: 10.1016/j.aej.2022.09.027.
Rabah F, Abukhaled M, Khuri S. Solution of a complex nonlinear fractional biochemical reaction model. Math Comput Appl, 2022;27, 45. doi: 10.3390/mca27030045.
Jamil A, Tu W, Ali S, Terriche Y, Guerrero J. Fractional-order PID controllers for temperature control: a review. Energies, 2022;15, 3800.
Jin B, Rundell W. A tutorial on inverse problems for anomalous diffusion processes. Inverse Probl. 2015;31, 035003. doi: 10.1088/0266-5611/31/3/035003.
Ortigueira MD, Tenreiro Machado J. Fractional signal processing and applications. Signal Process, 2003;83, 2285–2286. doi: 10.1016/s0165-1684(03)00181-6.
Zayernouri M, Matzavinos A. Fractional Adams–Bashforth/Moulton methods: an application to the fractional Keller–Segel chemotaxis system. J Comput Phys, 2016;317, 1–14. doi: 10.1016/j.jcp.2016.04.041.
Zabidi N, Majid Z, Kilicman A, Ibrahim Z. Numerical solution of fractional differential equations with Caputo derivative by using numerical fractional predict–correct technique. Adv Contin Discrete Models. 2022;26, 1–23. doi: 10.1186/s13662-022-03697-6.
Han C, Wang YL, Li ZY. A high-precision numerical approach to solving space fractional Gray-Scott model. Appl Math Lett, 2022;125, 107759. doi: 10.1016/j.aml.2021.107759.
Youssri YH, Atta A. Spectral Collocation Approach via Normalized Shifted Jacobi Polynomials for the Nonlinear Lane-Emden Equation with Fractal-Fractional Derivative. Fractal Fractional, 2023;7, 133. doi: 10.3390/fractalfract7020133.
Shoaib M, Abukhaled M, Kainat S, Nisar KS, Raja MA, Zubair G. Integrated Neuro-Evolution-Based Computing Paradigm to Study the COVID-19 Transposition and Severity in Romania and Pakistan. International Journal of Computational Intelligence Systems, 2022;15, 80. doi: 10.1007/s44196-022-00133-1.
Weera W,Botmart T, La-inchua T, et al. A stochastic computational scheme for the computer epidemic virus with delay effects. AIMS Math, 2023;8, 148–63. doi: 10.3934/math.2023007.
Rabah F, Abukhaled M, Khuri S. Solution of a complex nonlinear fractional biochemical reaction model. Mathematical and Computational Applications, 2022;27, 45. doi: 10.3390/mca27030045.
Qu H, She Z, Liu X. Homotopy Analysis Method for Three Types of Fractional Partial Differential Equations. Complexity, 2020;2020, 7232907. doi: 10.1155/2020/7232907.
Javeed S, Baleanu D, Waheed A, Shaukat Khan M, Affan H. Analysis of Homotopy Perturbation Method for Solving Fractional Order Differential Equations. Mathematics, 2019;7, 40. doi: 10.3390/math7010040.
Abukhaled M, Khuri S, Rabah F. Solution of a nonlinear fractional COVID-19 model. Int J Numer Methods Heat Fluid Flow, 2022;32, 3657–3670. doi: 10.1108/hff-01-2022-0042.
Abukhaled M, Khuri S. RLC electric circuit model of fractional order: a Green’s function approach. Int J Comput Math, 2023; doi: 10.1080/00207160.2023.2203787.
Abu Arqub O, Tayebi S, Momani S, Abukhaled M. Adaptation of the Novel Cubic B-Spline Algorithm for Dealing with Conformable Systems of Differential Boundary Value Problems concerning Two Points and Two Fractional Parameters. J Funct Spaces, 2023; 5322092. doi: 10.1155/2023/5322092.
El-Ajou A, Abu Arqub O, Al Zhour Z, Momani S. New results on fractional power series: theories and applications. Entropy, 2013;15, 5305–5323. doi: 10.3390/e15125305.
AbuArqubO. Application of residualpower seriesmethod for the solution of time-fractional Schrödinger equations in one-dimensional space. Fundamenta Informaticae, 2019;166, 87–110. doi: 10.3233/fi-2019-1795.
Majumder P. Anatomy of cornea. (Accessed 14 December 2023). Available from: https://www.eophtha.com/posts/anatomy-of-cornea.
Grosvenor T. Primary Care Optometry. 5th ed. Butterworth-Heinemann, 2007; 22–302.
O’Hara M. Clinical Ophthalmology: A Systemic Approach. 5th ed. Butterworth-Heinemann, 2004;
Seitz B, Langenbucher A, Zagrada D, Budde W, Kus M. Corneal dimensions in patients with various types of corneal dystrophies and their impact on penetrating keratoplasty. Klin Monatsbl Augenheilkd. 2000;217, 152–158. doi: 10.1055/S-2000-10338.
Denniston A, Murray P. Oxford Handbook of Ophthalmology. 2nd ed. Oxford University Press, 2009;
Troilo D. Neonatal eye growth and emmetropization-a literature review. Eye, 1992;6(2): 154–160. doi: 10.1038/eye.1992.31.
Grosvenor T, Scott R. Role of the axial length/corneal radius ratio in determining the refractive state of the eye. Optometry and Vision Science, 1994;71(9): 573–579. doi: 10.1097/00006324-199409000-00005.
Waltman SR, Hart MW. The cornea. in Adler’s Physiology of the Eye-Clinical Application. 8th ed. CV Mosby Coy, USA, 1987; 36–59.
Iyamu E, Iyamu J, Obiakor C. The role of axial length-corneal radius of curvature ratio in refractive state categorization in a Nigerian population. International Scholarly Research Notices, 2011; doi: 10.5402/2011/138941.
Okrasiński W, Plociniczak L. A nonlinear mathematical model of the corneal shape. Nonlinear Anal. Real World Appl. 2012;13, 1498–1505. doi: 0.1016/j.nonrwa.2011.11.014.
Plociniczak L, Okrasiński W, Nieto JJ, Dom´nguez O. On a nonlinear boundary value problem modeling corneal shape. J. Math. Anal. Appl. 2014;414, 461–471. doi: 10.1016/j.jmaa.2014.01.010.
He J. A remark on "A nonlinearmathematical model of the corneal shape". Nonlinear Anal. Real World Appl. 2012;13, 2863–2865. doi: 10.1016/j.nonrwa.2012.04.014.
Abukhaled M, Khuri S. An Efficient Semi-Analytical Solution of a One-Dimensional Curvature Equation that Describes the Human Corneal Shape. Math. Comput. Appl. 2019;24(8): doi: 10.3390/mca24010008.
Erturk V, Ahmadkhanlu A, Pushpendra K, Govidaraj V. Some novelmathematical analysis on a corneal shape model by using Caputo fractional derivative. Optik, 2022;261, 169086. doi: 10.1016/j.ijleo.2022.169086.